A novel critical velocity model for the incipient motion of non-spherical particles on cuttings bed in extended reach wells--颗粒学报PARTICUOLOGY

Hu, W., Guan, N., Zhang, J., Xu, B., & Zhu, H. (2025). A novel critical velocity model for the incipient motion of non-spherical particles on cuttings bed in extended reach wells. Particuology, 97, 193-206. https://doi.org/10.1016/j.partic.2024.12.011

A novel critical velocity model for the incipient motion of non-spherical particles on cuttings bed in extended reach wells

Wei Hu^a, Ning Guan^b, Jinya Zhang^a *, Binggui Xu^b, Hongwu Zhu^a

a College of Mechanical and Transportation Engineering, China University of Petroleum, Beijing, 102249, China
b CNPC Engineering Technology R&D Company Limited, Beijing, 102206, China

10.1016/j.partic.2024.12.011

Volume 97, February 2025, Pages 193-206

Received 10 October 2024, Revised 15 November 2024, Accepted 24 December 2024, Available online 9 January 2025, Version of Record 16 January 2025.

E-mail: zhjinya@163.com

Highlights

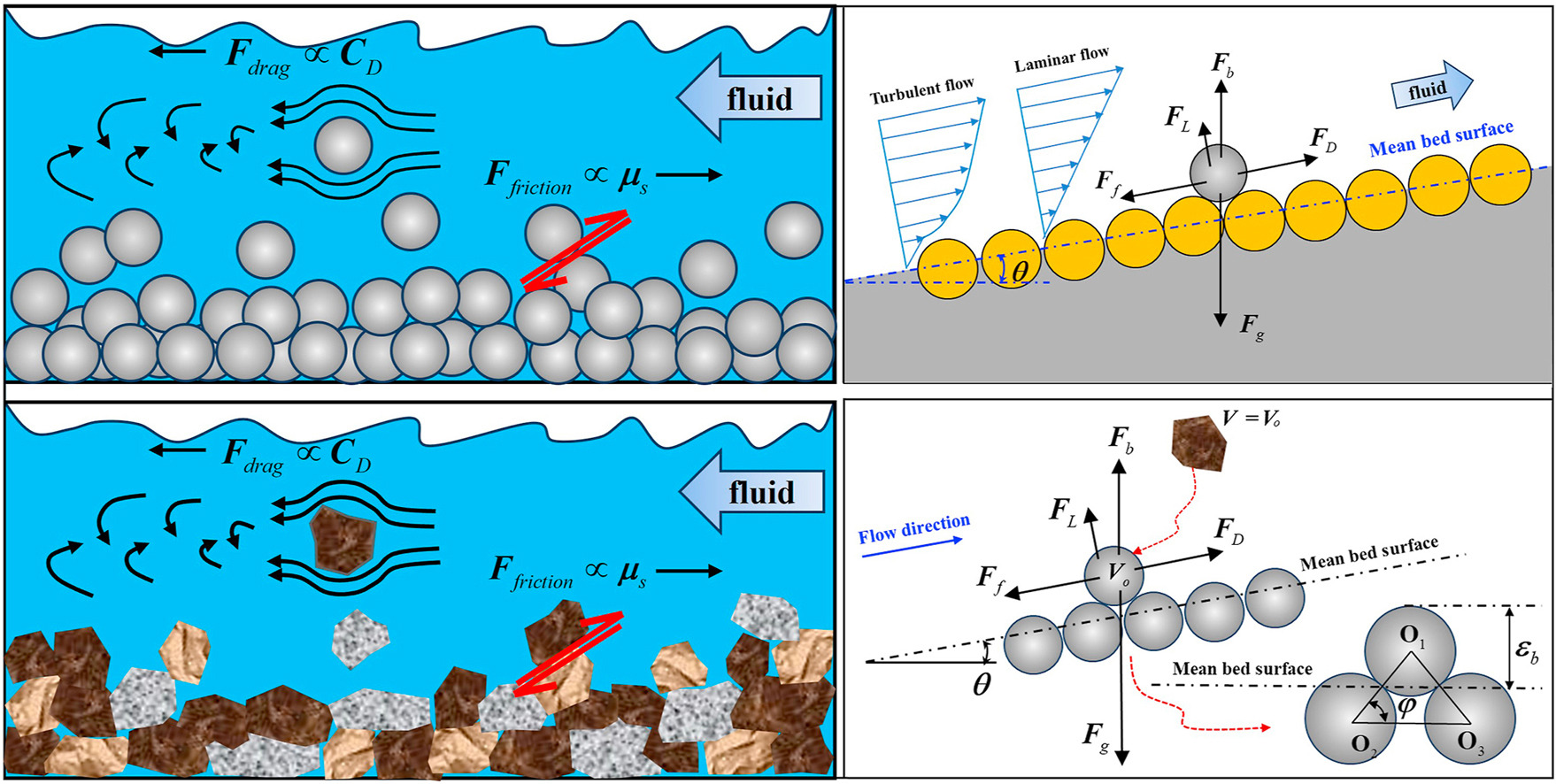
• Correction factors for shape-related drag and friction coefficients are established.

• A semi-mechanistic model for particle shape-related incipient motion criteria is proposed.

• A critical velocity calculation procedure for the incipient motion of particles is developed.

• The presented model is applicable to both Newtonian and non-Newtonian fluids.

Abstract

Accurately predicting the minimum velocity required to initiate particles movement on a cuttings bed surface during drilling operations is crucial for efficient and cost-effective removal of deposited particles. However, current models neglect the influence of particle shape on the drag coefficient and static friction coefficient during rolling and sliding on a cuttings bed. Accordingly, this study developed an experimental setup for cuttings transport and employed both theoretical analysis and experimental methods to investigate the critical velocity for the incipient motion of particles under various operational conditions. A novel semi-mechanical criterion model was developed for the incipient motion of particles, incorporating a shape correction factor for non-spherical particles. A balance equation for the threshold Shields number, determined by particle driving forces and resistances, was established, and a numerical procedure was formulated to determine the critical velocity for the incipient motion of particles. The model predictions show strong agreement with experimental results. The study found that higher eccentricity, inclination, and fluid viscosity increased the difficulty of initiating particle movement on the cuttings bed surface, thus requiring higher annular velocities for effective cuttings removal. Conversely, increasing particle size facilitated easier removal of the cuttings bed. Compared to non-Newtonian fluids, Newtonian fluids proved more effective in cuttings removal. The findings of this study are significant for optimizing hole cleaning parameters and improving the efficiency of cuttings removal.

Graphical abstract

Keywords

Cuttings transport; Critical velocity; Predictive model; Particle shape effect; Experimental study

文章导读